Betti number
In algebraic topology, the Betti numbers are used to distinguish topological spaces based on the connectivity of n-dimensional simplicial complexes. For the most reasonable finite-dimensional spaces (such as compact manifolds, finite simplicial complexes or CW complexes), the sequence of Betti numbers is 0 from some point onward (Betti numbers vanish above the dimension of a space), and they are all finite.
The nth Betti number represents the rank of the nth homology group, denoted Hn, which tells us the maximum number of cuts that can be made before separating a surface into two pieces or 0-cycles, 1-cycles, etc.[1] For example, if then , if then , if then , if then , etc. Note that only the ranks of infinite groups are considered, so for example if , where is the finite cyclic group of order 2, then . These finite components of the homology groups are their torsion subgroups, and they are denoted by torsion coefficients.
The term "Betti numbers" was coined by Henri Poincaré after Enrico Betti. The modern formulation is due to Emmy Noether. Betti numbers are used today in fields such as simplicial homology, computer science and digital images.
Informally, the kth Betti number refers to the number of k-dimensional holes on a topological surface. A "k-dimensional hole" is a k-dimensional cycle that is not a boundary of a (k+1)-dimensional object.
The first few Betti numbers have the following definitions for 0-dimensional, 1-dimensional, and 2-dimensional simplicial complexes:
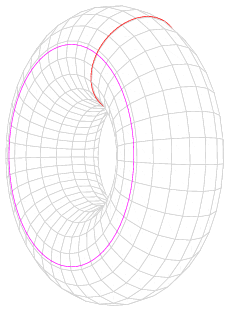
Thus, for example, a torus has one connected surface component so b0 = 1, two "circular" holes (one equatorial and one meridional) so b1 = 2, and a single cavity enclosed within the surface so b2 = 1.
Another interpretation of bk is the maximum number of k-dimensional curves that can be removed while the object remains connected. For example, the torus remains connected after removing two 1-dimensional curves (equatorial and meridional) so b1 = 2.[2]
The two-dimensional Betti numbers are easier to understand because we can see the world in 0, 1, 2, and 3-dimensions.
Formal definition[edit]
For a non-negative integer k, the kth Betti number bk(X) of the space X is defined as the rank (number of linearly independent generators) of the abelian group Hk(X), the kth homology group of X. The kth homology group is , the s are the boundary maps of the simplicial complex and the rank of Hk is the kth Betti number. Equivalently, one can define it as the vector space dimension of Hk(X; Q) since the homology group in this case is a vector space over Q. The universal coefficient theorem, in a very simple torsion-free case, shows that these definitions are the same.
More generally, given a field F one can define bk(X, F), the kth Betti number with coefficients in F, as the vector space dimension of Hk(X, F).
Poincaré polynomial[edit]
The Poincaré polynomial of a surface is defined to be the generating function of its Betti numbers. For example, the Betti numbers of the torus are 1, 2, and 1; thus its Poincaré polynomial is . The same definition applies to any topological space which has a finitely generated homology.
Given a topological space which has a finitely generated homology, the Poincaré polynomial is defined as the generating function of its Betti numbers, via the polynomial where the coefficient of is .
Examples[edit]
Betti numbers of a graph[edit]
Consider a topological graph G in which the set of vertices is V, the set of edges is E, and the set of connected components is C. As explained in the page on graph homology, its homology groups are given by:















