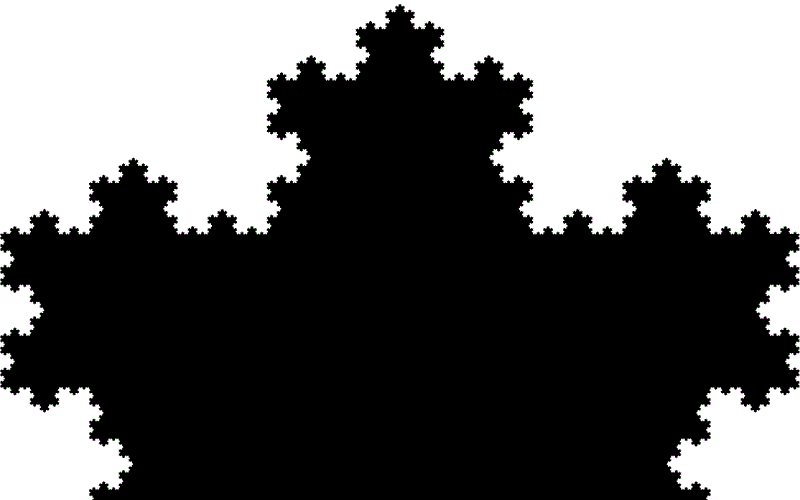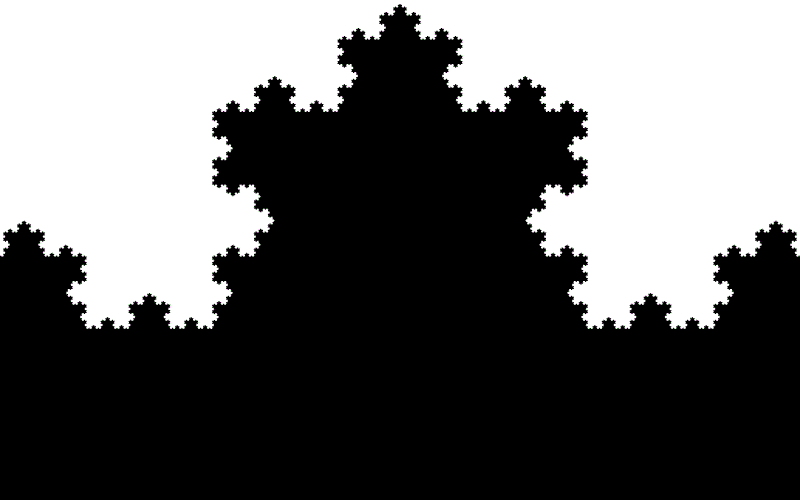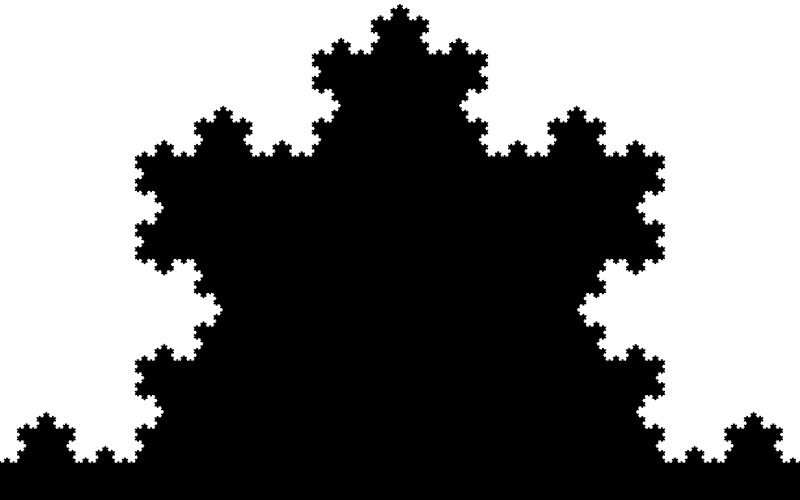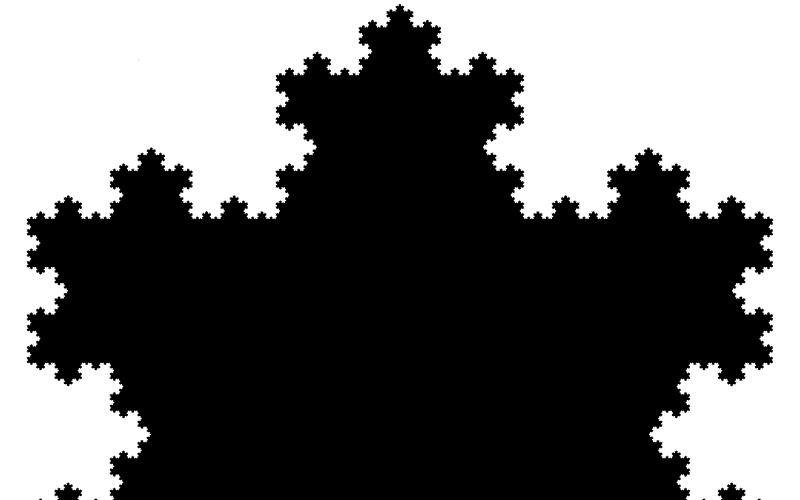
Self-similarity
In mathematics, a self-similar object is exactly or approximately similar to a part of itself (i.e., the whole has the same shape as one or more of the parts). Many objects in the real world, such as coastlines, are statistically self-similar: parts of them show the same statistical properties at many scales.[2] Self-similarity is a typical property of fractals. Scale invariance is an exact form of self-similarity where at any magnification there is a smaller piece of the object that is similar to the whole. For instance, a side of the Koch snowflake is both symmetrical and scale-invariant; it can be continually magnified 3x without changing shape. The non-trivial similarity evident in fractals is distinguished by their fine structure, or detail on arbitrarily small scales. As a counterexample, whereas any portion of a straight line may resemble the whole, further detail is not revealed.
A time developing phenomenon is said to exhibit self-similarity if the numerical value of certain observable quantity
measured at different times are different but the corresponding dimensionless quantity at given value of remain invariant. It happens if the quantity exhibits dynamic scaling. The idea is just an extension of the idea of similarity of two triangles.[3][4][5] Note that two triangles are similar if the numerical values of their sides are different however the corresponding dimensionless quantities, such as their angles, coincide.
Peitgen et al. explain the concept as such:
Since mathematically, a fractal may show self-similarity under indefinite magnification, it is impossible to recreate this physically. Peitgen et al. suggest studying self-similarity using approximations:
This vocabulary was introduced by Benoit Mandelbrot in 1964.[8]

