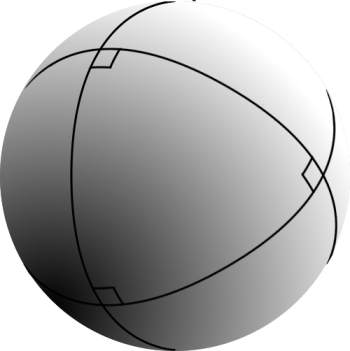
Katana VentraIP

Spherical trigonometry

Spherical trigonometry is the branch of spherical geometry that deals with the metrical relationships between the sides and angles of spherical triangles, traditionally expressed using trigonometric functions. On the sphere, geodesics are great circles. Spherical trigonometry is of great importance for calculations in astronomy, geodesy, and navigation.

The origins of spherical trigonometry in Greek mathematics and the major developments in Islamic mathematics are discussed fully in History of trigonometry and Mathematics in medieval Islam. The subject came to fruition in Early Modern times with important developments by John Napier, Delambre and others, and attained an essentially complete form by the end of the nineteenth century with the publication of Todhunter's textbook Spherical trigonometry for the use of colleges and Schools.[1] Since then, significant developments have been the application of vector methods, quaternion methods, and the use of numerical methods.

Both vertices and angles at the vertices of a triangle are denoted by the same upper case letters A, B, and C.

Sides are denoted by lower-case letters: a, b, and c. The sphere has a radius of 1, and so the side lengths and lower case angles are equivalent (see arc length).

The angle A (respectively, B and C) may be regarded either as the angle between the two planes that intersect the sphere at the vertex A, or, equivalently, as the angle between the of the great circle arcs where they meet at the vertex.

tangents

Angles are expressed in . The angles of proper spherical triangles are (by convention) less than π, so that

(Todhunter,[1] Art.22,32).

radians

Identities[edit]

Supplemental cosine rules[edit]

Applying the cosine rules to the polar triangle gives (Todhunter,[1] Art.47), i.e. replacing A by π – a, a by π – A etc.,

Case 1: three sides given (SSS). The cosine rule may be used to give the angles A, B, and C but, to avoid ambiguities, the half angle formulae are preferred.

Case 2: two sides and an included angle given (SAS). The cosine rule gives a and then we are back to Case 1.

Case 3: two sides and an opposite angle given (SSA). The sine rule gives C and then we have Case 7. There are either one or two solutions.

Case 4: two angles and an included side given (ASA). The four-part cotangent formulae for sets (cBaC) and (BaCb) give c and b, then A follows from the sine rule.

Case 5: two angles and an opposite side given (AAS). The sine rule gives b and then we have Case 7 (rotated). There are either one or two solutions.

Case 6: three angles given (AAA). The supplemental cosine rule may be used to give the sides a, b, and c but, to avoid ambiguities, the half-side formulae are preferred.

Case 7: two angles and two opposite sides given (SSAA). Use Napier's analogies for a and A; or, use Case 3 (SSA) or case 5 (AAS).

Air navigation

Celestial navigation

Ellipsoidal trigonometry

or spherical distance

Great-circle distance

Lenart sphere

Schwarz triangle

Spherical geometry

Spherical polyhedron

Triangulation (surveying)

"Spherical Trigonometry". MathWorld. a more thorough list of identities, with some derivation

Weisstein, Eric W.

"Spherical Triangle". MathWorld. a more thorough list of identities, with some derivation

Weisstein, Eric W.

A free software to solve the spherical triangles, configurable to different practical applications and configured for gnomonic

TriSph

by Sudipto Banerjee. The paper derives the spherical law of cosines and law of sines using elementary linear algebra and projection matrices.

"Revisiting Spherical Trigonometry with Orthogonal Projectors"

. Wolfram Demonstrations Project. by Okay Arik

"A Visual Proof of Girard's Theorem"

a manuscript in Arabic that dates back to 1740 and talks about spherical trigonometry, with diagrams

"The Book of Instruction on Deviant Planes and Simple Planes"

Robert G. Chamberlain, William H. Duquette, Jet Propulsion Laboratory. The paper develops and explains many useful formulae, perhaps with a focus on navigation and cartography.

Some Algorithms for Polygons on a Sphere

Online computation of spherical triangles