Bernoulli's principle

Bernoulli's principle is a key concept in fluid dynamics that relates pressure, speed and height. Bernoulli's principle states that an increase in the speed of a parcel of fluid occurs simultaneously with a decrease in either the pressure or the height above a datum.^[1]^: Ch.3^[2]^{: 156–164, § 3.5} The principle is named after the Swiss mathematician and physicist Daniel Bernoulli, who published it in his book Hydrodynamica in 1738.^[3] Although Bernoulli deduced that pressure decreases when the flow speed increases, it was Leonhard Euler in 1752 who derived Bernoulli's equation in its usual form.^[4]^[5]

This article is about Bernoulli's principle and Bernoulli's equation in fluid dynamics. For Bernoulli's theorem in probability, see law of large numbers. For an unrelated topic in ordinary differential equations, see Bernoulli differential equation.

Bernoulli's principle can be derived from the principle of conservation of energy. This states that, in a steady flow, the sum of all forms of energy in a fluid is the same at all points that are free of viscous forces. This requires that the sum of kinetic energy, potential energy and internal energy remains constant.^[2]^{: § 3.5} Thus an increase in the speed of the fluid—implying an increase in its kinetic energy—occurs with a simultaneous decrease in (the sum of) its potential energy (including the static pressure) and internal energy. If the fluid is flowing out of a reservoir, the sum of all forms of energy is the same because in a reservoir the energy per unit volume (the sum of pressure and gravitational potential $ρ g h$ ) is the same everywhere.^[6]^{: Example 3.5 and p.116}

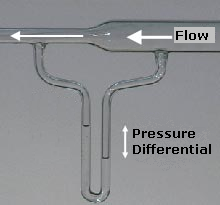
Bernoulli's principle can also be derived directly from Isaac Newton's second Law of Motion. If a small volume of fluid is flowing horizontally from a region of high pressure to a region of low pressure, then there is more pressure behind than in front. This gives a net force on the volume, accelerating it along the streamline.^[a]^[b]^[c]

Fluid particles are subject only to pressure and their own weight. If a fluid is flowing horizontally and along a section of a streamline, where the speed increases it can only be because the fluid on that section has moved from a region of higher pressure to a region of lower pressure; and if its speed decreases, it can only be because it has moved from a region of lower pressure to a region of higher pressure. Consequently, within a fluid flowing horizontally, the highest speed occurs where the pressure is lowest, and the lowest speed occurs where the pressure is highest.^[10]

Bernoulli's principle is only applicable for isentropic flows: when the effects of irreversible processes (like turbulence) and non-adiabatic processes (e.g. thermal radiation) are small and can be neglected. However, the principle can be applied to various types of flow within these bounds, resulting in various forms of Bernoulli's equation. The simple form of Bernoulli's equation is valid for incompressible flows (e.g. most liquid flows and gases moving at low Mach number). More advanced forms may be applied to compressible flows at higher Mach numbers.

$v$ is the fluid flow at a point,

speed

$g$ is the ,

acceleration due to gravity

$z$ is the of the point above a reference plane, with the positive $z$ -direction pointing upward—so in the direction opposite to the gravitational acceleration,

elevation

$p$ is the at the chosen point, and

pressure

$\rho$ is the of the fluid at all points in the fluid.

density

$p$ is the pressure

$ρ$ is the density and $ρ (p)$ indicates that it is a function of pressure

$v$ is the flow speed

$Ψ$ is the potential associated with the conservative force field, often the

gravitational potential

Bernoulli's principle can be used to calculate the lift force on an , if the behaviour of the fluid flow in the vicinity of the foil is known. For example, if the air flowing past the top surface of an aircraft wing is moving faster than the air flowing past the bottom surface, then Bernoulli's principle implies that the pressure on the surfaces of the wing will be lower above than below. This pressure difference results in an upwards lifting force.^[d]^[23] Whenever the distribution of speed past the top and bottom surfaces of a wing is known, the lift forces can be calculated (to a good approximation) using Bernoulli's equations,^[24] which were established by Bernoulli over a century before the first man-made wings were used for the purpose of flight.

airfoil

The used in many reciprocating engines contains a venturi to create a region of low pressure to draw fuel into the carburetor and mix it thoroughly with the incoming air. The low pressure in the throat of a venturi can be explained by Bernoulli's principle; in the narrow throat, the air is moving at its fastest speed and therefore it is at its lowest pressure.

carburetor

An on a steam locomotive or a static boiler.

injector

The and static port on an aircraft are used to determine the airspeed of the aircraft. These two devices are connected to the airspeed indicator, which determines the dynamic pressure of the airflow past the aircraft. Bernoulli's principle is used to calibrate the airspeed indicator so that it displays the indicated airspeed appropriate to the dynamic pressure.^[1]^{: § 3.8}

pitot tube

A utilizes Bernoulli's principle to create a force by turning pressure energy generated by the combustion of propellants into velocity. This then generates thrust by way of Newton's third law of motion.

De Laval nozzle

The flow speed of a fluid can be measured using a device such as a Venturi meter or an , which can be placed into a pipeline to reduce the diameter of the flow. For a horizontal device, the continuity equation shows that for an incompressible fluid, the reduction in diameter will cause an increase in the fluid flow speed. Subsequently, Bernoulli's principle then shows that there must be a decrease in the pressure in the reduced diameter region. This phenomenon is known as the Venturi effect.

orifice plate

The maximum possible drain rate for a tank with a hole or tap at the base can be calculated directly from Bernoulli's equation and is found to be proportional to the square root of the height of the fluid in the tank. This is , which is compatible with Bernoulli's principle. Increased viscosity lowers this drain rate; this is reflected in the discharge coefficient, which is a function of the Reynolds number and the shape of the orifice.^[25]

Torricelli's law

The relies on this principle to create a non-contact adhesive force between a surface and the gripper.

Bernoulli grip

During a match, bowlers continually polish one side of the ball. After some time, one side is quite rough and the other is still smooth. Hence, when the ball is bowled and passes through air, the speed on one side of the ball is faster than on the other, and this results in a pressure difference between the sides; this leads to the ball rotating ("swinging") while travelling through the air, giving advantage to the bowlers.

cricket

In modern everyday life there are many observations that can be successfully explained by application of Bernoulli's principle, even though no real fluid is entirely inviscid,^[22] and a small viscosity often has a large effect on the flow.

Torricelli's law

Coandă effect

– for the flow of an inviscid fluid

Euler equations

– applied fluid mechanics for liquids

Hydraulics

– for the flow of a viscous fluid

Navier–Stokes equations

Teapot effect

Terminology in fluid dynamics

The Flow of Dry Water - The Feynman Lectures on Physics

Science 101 Q: Is It Really Caused by the Bernoulli Effect?

Bernoulli equation calculator

Millersville University – Applications of Euler's equation

Archived 2012-07-15 at the Wayback Machine

NASA – Beginner's guide to aerodynamics

Archived 2012-02-08 at the Wayback Machine

Bernoulli's principle

speed

acceleration due to gravity

elevation

pressure

density

gravitational potential

airfoil

carburetor

injector

pitot tube

De Laval nozzle

orifice plate

Torricelli's law

Bernoulli grip

cricket

Torricelli's law

Coandă effect

Euler equations

Hydraulics

Navier–Stokes equations

Teapot effect

Terminology in fluid dynamics

The Flow of Dry Water - The Feynman Lectures on Physics

Science 101 Q: Is It Really Caused by the Bernoulli Effect?

Bernoulli equation calculator

Millersville University – Applications of Euler's equation

NASA – Beginner's guide to aerodynamics

Misinterpretations of Bernoulli's equation – Weltner and Ingelman-Sundberg