Riemann hypothesis

In mathematics, the Riemann hypothesis is the conjecture that the Riemann zeta function has its zeros only at the negative even integers and complex numbers with real part 1/2. Many consider it to be the most important unsolved problem in pure mathematics.^[1] It is of great interest in number theory because it implies results about the distribution of prime numbers. It was proposed by Bernhard Riemann (1859), after whom it is named.

For the musical term, see Riemannian theory.

The Riemann hypothesis and some of its generalizations, along with Goldbach's conjecture and the twin prime conjecture, make up Hilbert's eighth problem in David Hilbert's list of twenty-three unsolved problems; it is also one of the Clay Mathematics Institute's Millennium Prize Problems, which offers US$1 million to anyone who solves any of them. The name is also used for some closely related analogues, such as the Riemann hypothesis for curves over finite fields.

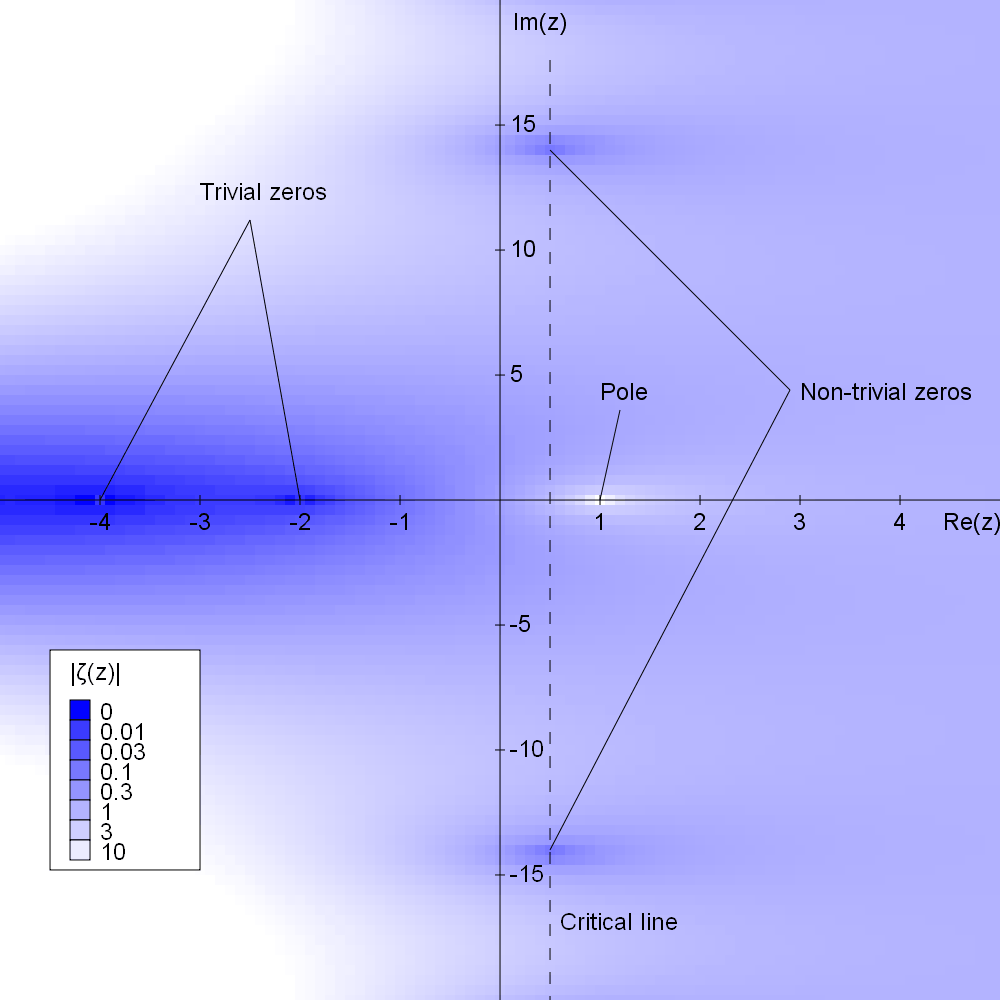
The Riemann zeta function ζ(s) is a function whose argument s may be any complex number other than 1, and whose values are also complex. It has zeros at the negative even integers; that is, ζ(s) = 0 when s is one of −2, −4, −6, .... These are called its trivial zeros. The zeta function is also zero for other values of s, which are called nontrivial zeros. The Riemann hypothesis is concerned with the locations of these nontrivial zeros, and states that:

Thus, if the hypothesis is correct, all the nontrivial zeros lie on the critical line consisting of the complex numbers 1/2 + i t, where t is a real number and i is the imaginary unit.

Riemann zeta function along the critical line with Re(s) = 1/2. Real values are shown on the horizontal axis and imaginary values are on the vertical axis. Re(ζ(1/2 + it)), Im(ζ(1/2 + it)) is plotted with t ranging between −30 and 30.^[3]

Animation showing in 3D the Riemann zeta function critical strip (blue, where s has real part between 0 and 1), critical line (red, for real part of s equals 0.5) and zeroes (cross between red and orange): [x,y,z] = [Re(ζ(r + it)), Im(ζ(r + it)), t] with 0.1 ≤ r ≤ 0.9 and 1 ≤ t ≤ 51

The real part (red) and imaginary part (blue) of the Riemann zeta function ζ(s) along the critical line in the complex plane with real part Re(s) = 1/2. The first nontrivial zeros, where ζ(s) equals zero, occur where both curves touch the horizontal x-axis, for complex numbers with imaginary parts Im(s) equaling ±14.135, ±21.022 and ±25.011.

The Riemann zeta function is defined for complex s with real part greater than 1 by the absolutely convergent infinite series

Leonhard Euler already considered this series in the 1730s for real values of s, in conjunction with his solution to the Basel problem. He also proved that it equals the Euler product

where the infinite product extends over all prime numbers p.^[2]

The Riemann hypothesis discusses zeros outside the region of convergence of this series and Euler product. To make sense of the hypothesis, it is necessary to analytically continue the function to obtain a form that is valid for all complex s. Because the zeta function is meromorphic, all choices of how to perform this analytic continuation will lead to the same result, by the identity theorem. A first step in this continuation observes that the series for the zeta function and the Dirichlet eta function satisfy the relation

within the region of convergence for both series. However, the zeta function series on the right converges not just when the real part of s is greater than one, but more generally whenever s has positive real part. Thus, the zeta function can be redefined as $\eta (s)/(1-2/2^{s})$ , extending it from Re(s) > 1 to a larger domain: Re(s) > 0, except for the points where $1-2/2^{s}$ is zero. These are the points $s=1+2\pi in/\log 2$ where $n$ can be any nonzero integer; the zeta function can be extended to these values too by taking limits (see Dirichlet eta function § Landau's problem with ζ(s) = η(s)/0 and solutions), giving a finite value for all values of s with positive real part except for the simple pole at s = 1.

In the strip 0 < Re(s) < 1 this extension of the zeta function satisfies the functional equation

One may then define ζ(s) for all remaining nonzero complex numbers s (Re(s) ≤ 0 and s ≠ 0) by applying this equation outside the strip, and letting ζ(s) equal the right-hand side of the equation whenever s has non-positive real part (and s ≠ 0).

If s is a negative even integer then ζ(s) = 0 because the factor sin( $π$ s/2) vanishes; these are the trivial zeros of the zeta function. (If s is a positive even integer this argument does not apply because the zeros of the sine function are cancelled by the poles of the gamma function as it takes negative integer arguments.)

The value ζ(0) = −1/2 is not determined by the functional equation, but is the limiting value of ζ(s) as s approaches zero. The functional equation also implies that the zeta function has no zeros with negative real part other than the trivial zeros, so all non-trivial zeros lie in the critical strip where s has real part between 0 and 1.

In 1913, showed that the generalized Riemann hypothesis implies that Gauss's list of imaginary quadratic fields with class number 1 is complete, though Baker, Stark and Heegner later gave unconditional proofs of this without using the generalized Riemann hypothesis.

Grönwall

In 1917, Hardy and Littlewood showed that the generalized Riemann hypothesis implies a conjecture of Chebyshev that $\lim _{x\to 1^{-}}\sum _{p>2}(-1)^{(p+1)/2}x^{p}=+\infty ,$ which says that primes 3 mod 4 are more common than primes 1 mod 4 in some sense. (For related results, see .)

Prime number theorem § Prime number race

In 1923, Hardy and Littlewood showed that the generalized Riemann hypothesis implies a weak form of the for odd numbers: that every sufficiently large odd number is the sum of three primes, though in 1937 Vinogradov gave an unconditional proof. In 1997 Deshouillers, Effinger, te Riele, and Zinoviev showed that the generalized Riemann hypothesis implies that every odd number greater than 5 is the sum of three primes. In 2013 Harald Helfgott proved the ternary Goldbach conjecture without the GRH dependence, subject to some extensive calculations completed with the help of David J. Platt.

Goldbach conjecture

In 1934, Chowla showed that the generalized Riemann hypothesis implies that the first prime in the arithmetic progression a mod m is at most Km²log(m)² for some fixed constant K.

In 1967, Hooley showed that the generalized Riemann hypothesis implies .

Artin's conjecture on primitive roots

In 1973, Weinberger showed that the generalized Riemann hypothesis implies that Euler's list of is complete.

idoneal numbers

showed that the generalized Riemann hypothesis for the zeta functions of all algebraic number fields implies that any number field with class number 1 is either Euclidean or an imaginary quadratic number field of discriminant −19, −43, −67, or −163.

Weinberger (1973)

In 1976, G. Miller showed that the generalized Riemann hypothesis implies that one can in polynomial time via the Miller test. In 2002, Manindra Agrawal, Neeraj Kayal and Nitin Saxena proved this result unconditionally using the AKS primality test.

test if a number is prime

discussed how the generalized Riemann hypothesis can be used to give sharper estimates for discriminants and class numbers of number fields.

Odlyzko (1990)

showed that the generalized Riemann hypothesis implies that Ramanujan's integral quadratic form x² + y² + 10z² represents all integers that it represents locally, with exactly 18 exceptions.

Ono & Soundararajan (1997)

In 2021, Alexander (Alex) Dunn and proved Patterson's conjecture on cubic Gauss sums, under the assumption of the GRH.^[18]^[19]

Maksym Radziwill

Generalizations and analogs[edit]

Dirichlet L-series and other number fields[edit]

The Riemann hypothesis can be generalized by replacing the Riemann zeta function by the formally similar, but much more general, global L-functions. In this broader setting, one expects the non-trivial zeros of the global L-functions to have real part 1/2. It is these conjectures, rather than the classical Riemann hypothesis only for the single Riemann zeta function, which account for the true importance of the Riemann hypothesis in mathematics.

The generalized Riemann hypothesis extends the Riemann hypothesis to all Dirichlet L-functions. In particular it implies the conjecture that Siegel zeros (zeros of L-functions between 1/2 and 1) do not exist.

The extended Riemann hypothesis extends the Riemann hypothesis to all Dedekind zeta functions of algebraic number fields. The extended Riemann hypothesis for abelian extension of the rationals is equivalent to the generalized Riemann hypothesis. The Riemann hypothesis can also be extended to the L-functions of Hecke characters of number fields.

The grand Riemann hypothesis extends it to all automorphic zeta functions, such as Mellin transforms of Hecke eigenforms.

Function fields and zeta functions of varieties over finite fields[edit]

Artin (1924) introduced global zeta functions of (quadratic) function fields and conjectured an analogue of the Riemann hypothesis for them, which has been proved by Hasse in the genus 1 case and by Weil (1948) in general. For instance, the fact that the Gauss sum, of the quadratic character of a finite field of size q (with q odd), has absolute value ${\sqrt {q}}$ is actually an instance of the Riemann hypothesis in the function field setting. This led Weil (1949) to conjecture a similar statement for all algebraic varieties; the resulting Weil conjectures were proved by Pierre Deligne (1974, 1980).

Arithmetic zeta functions of arithmetic schemes and their L-factors[edit]

Arithmetic zeta functions generalise the Riemann and Dedekind zeta functions as well as the zeta functions of varieties over finite fields to every arithmetic scheme or a scheme of finite type over integers. The arithmetic zeta function of a regular connected equidimensional arithmetic scheme of Kronecker dimension n can be factorized into the product of appropriately defined L-factors and an auxiliary factor Jean-Pierre Serre (1969–1970). Assuming a functional equation and meromorphic continuation, the generalized Riemann hypothesis for the L-factor states that its zeros inside the critical strip $\Re (s)\in (0,n)$ lie on the central line. Correspondingly, the generalized Riemann hypothesis for the arithmetic zeta function of a regular connected equidimensional arithmetic scheme states that its zeros inside the critical strip lie on vertical lines $\Re (s)=1/2,3/2,\dots ,n-1/2$ and its poles inside the critical strip lie on vertical lines $\Re (s)=1,2,\dots ,n-1$ . This is known for schemes in positive characteristic and follows from Pierre Deligne (1974, 1980), but remains entirely unknown in characteristic zero.

Location of the zeros[edit]

Number of zeros[edit]

The functional equation combined with the argument principle implies that the number of zeros of the zeta function with imaginary part between 0 and T is given by

Several analogues of the Riemann hypothesis have already been proved. The proof of the Riemann hypothesis for varieties over finite fields by is possibly the single strongest theoretical reason in favor of the Riemann hypothesis. This provides some evidence for the more general conjecture that all zeta functions associated with automorphic forms satisfy a Riemann hypothesis, which includes the classical Riemann hypothesis as a special case. Similarly Selberg zeta functions satisfy the analogue of the Riemann hypothesis, and are in some ways similar to the Riemann zeta function, having a functional equation and an infinite product expansion analogous to the Euler product expansion. But there are also some major differences; for example, they are not given by Dirichlet series. The Riemann hypothesis for the Goss zeta function was proved by Sheats (1998). In contrast to these positive examples, some Epstein zeta functions do not satisfy the Riemann hypothesis even though they have an infinite number of zeros on the critical line.^[13] These functions are quite similar to the Riemann zeta function, and have a Dirichlet series expansion and a functional equation, but the ones known to fail the Riemann hypothesis do not have an Euler product and are not directly related to automorphic representations.

Deligne (1974)

At first, the numerical verification that many zeros lie on the line seems strong evidence for it. But analytic number theory has had many conjectures supported by substantial numerical evidence that turned out to be false. See for a notorious example, where the first exception to a plausible conjecture related to the Riemann hypothesis probably occurs around 10³¹⁶; a counterexample to the Riemann hypothesis with imaginary part this size would be far beyond anything that can currently be computed using a direct approach. The problem is that the behavior is often influenced by very slowly increasing functions such as log log T, that tend to infinity, but do so so slowly that this cannot be detected by computation. Such functions occur in the theory of the zeta function controlling the behavior of its zeros; for example the function S(T) above has average size around (log log T)^1/2. As S(T) jumps by at least 2 at any counterexample to the Riemann hypothesis, one might expect any counterexamples to the Riemann hypothesis to start appearing only when S(T) becomes large. It is never much more than 3 as far as it has been calculated, but is known to be unbounded, suggesting that calculations may not have yet reached the region of typical behavior of the zeta function.

Skewes number

's probabilistic argument for the Riemann hypothesis^[33] is based on the observation that if μ(x) is a random sequence of "1"s and "−1"s then, for every ε > 0, the partial sums $M(x)=\sum _{n\leq x}\mu (n)$ (the values of which are positions in a simple random walk) satisfy the bound $M(x)=O(x^{1/2+\varepsilon })$ with probability 1. The Riemann hypothesis is equivalent to this bound for the Möbius function μ and the Mertens function M derived in the same way from it. In other words, the Riemann hypothesis is in some sense equivalent to saying that μ(x) behaves like a random sequence of coin tosses. When μ(x) is nonzero its sign gives the parity of the number of prime factors of x, so informally the Riemann hypothesis says that the parity of the number of prime factors of an integer behaves randomly. Such probabilistic arguments in number theory often give the right answer, but tend to be very hard to make rigorous, and occasionally give the wrong answer for some results, such as Maier's theorem.

Denjoy

The calculations in show that the zeros of the zeta function behave very much like the eigenvalues of a random Hermitian matrix, suggesting that they are the eigenvalues of some self-adjoint operator, which would imply the Riemann hypothesis. All attempts to find such an operator have failed.

Odlyzko (1987)

There are several theorems, such as for sufficiently large odd numbers, that were first proved using the generalized Riemann hypothesis, and later shown to be true unconditionally. This could be considered as weak evidence for the generalized Riemann hypothesis, as several of its "predictions" are true.

Goldbach's weak conjecture

,^[34] where two zeros are sometimes very close, is sometimes given as a reason to disbelieve the Riemann hypothesis. But one would expect this to happen occasionally by chance even if the Riemann hypothesis is true, and Odlyzko's calculations suggest that nearby pairs of zeros occur just as often as predicted by Montgomery's conjecture.

Lehmer's phenomenon

suggests that the most compelling reason for the Riemann hypothesis for most mathematicians is the hope that primes are distributed as regularly as possible.^[35]

Patterson

Mathematical papers about the Riemann hypothesis tend to be cautiously noncommittal about its truth. Of authors who express an opinion, most of them, such as Riemann (1859) and Bombieri (2000), imply that they expect (or at least hope) that it is true. The few authors who express serious doubt about it include Ivić (2008), who lists some reasons for skepticism, and Littlewood (1962), who flatly states that he believes it false, that there is no evidence for it and no imaginable reason it would be true. The consensus of the survey articles (Bombieri 2000, Conrey 2003, and Sarnak 2005) is that the evidence for it is strong but not overwhelming, so that while it is probably true there is reasonable doubt.

Some of the arguments for and against the Riemann hypothesis are listed by Sarnak (2005), Conrey (2003), and Ivić (2008), and include the following:

(2003a), The greatest unsolved problem in mathematics, Farrar, Straus and Giroux, New York, ISBN 978-0-374-25007-2, MR 1979664

Sabbagh, Karl

(2003b), Dr. Riemann's zeros, Atlantic Books, London, ISBN 978-1-843-54101-1

Sabbagh, Karl

(2003), The music of the primes, HarperCollins Publishers, ISBN 978-0-06-621070-4, MR 2060134

du Sautoy, Marcus

Rockmore, Dan (2005), , Pantheon Books, ISBN 978-0-375-42136-5, MR 2269393

Stalking the Riemann hypothesis

(2003), Prime Obsession, Joseph Henry Press, Washington, DC, ISBN 978-0-309-08549-6, MR 1968857

Derbyshire, John

Watkins, Matthew (2015), , Liberalis Books, ISBN 978-1782797814, MR 0000000

Mystery of the Prime Numbers

(2014), The Riemann Hypothesis Numberphile, Mar 11, 2014 (video)

Frenkel, Edward

(2021). In Pursuit of Zeta-3: The World's Most Mysterious Unsolved Math Problem. Princeton University Press. ISBN 978-0691206073.

Nahin, Paul J.

Media related to Riemann hypothesis at Wikimedia Commons